A second way to estimate betas is to look at the fundamentals of the business. The beta for a firm may be estimated from a regression but it is determined by decisions the firm has made on what business to be in, how much operating leverage to use in the business and by the degree to which the firm uses financial leverage. This approach is less reliant on historical betas and more cognisant of their fundamental determinants.

The beta of a firm is determined by three variables -

1. the type of business or businesses the firm is in,
2. the degree of operating leverage of the firm and
3. the firm's financial leverage.

Since betas measure the risk of a firm relative to a market index, the more sensitive a business is to market conditions, the higher its beta. Thus, other things remaining equal, cyclical firms can be expected to have higher betas than non-cyclical firms. Companies involved in housing and automobiles, two sectors of the economy which are very sensitive to economic conditions, should have higher betas than companies in food processing and tobacco, which are relatively insensitive to business cycles.

This view can be extended to a company's products. The degree to which a product's purchase is discretionary will affect the beta of the firm manufacturing the product. Firms whose products are much more discretionary to their customers should have higher betas than firms whose products are viewed as necessary or less discretionary. Thus, the beta of Procter and Gamble, which sells daily household products, should be lower than the beta of Gucci, which manufactures luxury products.

The degree of operating leverage is a function of the cost structure of a firm and is usually defined in terms of the relationship between fixed costs and total costs. A firm that has high fixed costs relative to total costs is said to have high operating leverage. A firm with high operating leverage will also have higher variability in operating income than would a firm producing a similar product with low operating leverage. Other things remaining equal, the higher variance in operating income will lead to a higher beta for the firm with high operating leverage.

Can firms change their operating leverage? While some of a firm's cost structure is determined by the business it is in (an energy utility has to build expensive power plants and airlines have to lease expensive planes), firms in the United States have become increasingly inventive in lowering the fixed cost component in their total costs. For instance, firms have made cost structures more flexible by

• negotiating labour contracts that emphasise flexibility and allow the firm to make its
• labour costs more sensitive to its financial success;
• entering into joint venture agreements, where the fixed costs are borne or shared by someone else; and
• sub-contracting manufacturing and outsourcing, which reduce the need for expensive plant and equipment.

While operating leverage affects betas, it is difficult to measure the operating leverage of a firm, at least from the outside, since fixed and variable costs are often aggregated in income statements. It is possible to get an approximate measure of the operating leverage of a firm by looking at changes in operating income as a function of changes in sales.

Degree of Operating leverage = % Change in Operating Profit / % Change in Sales

Generally, smaller firms with higher growth potential are viewed as riskier than larger, more stable firms. While the rationale for this argument is clear when talking about total risk, it becomes more difficult to see when looking at market risk or betas. Should a smaller software firm have a higher beta than a larger software firm? One reason to believe that it should is operating leverage.
If there is a set-up cost associated with investing in infrastructure or economies of scale, smaller firms will have higher fixed costs than larger firms, leading in turn to higher betas for these firms.
With growth firms, the argument for higher betas rests on the notion of discretionary versus non-discretionary purchases. For a high growth firm to deliver on its growth, new customers have to adopt the product or existing customers have to buy more of the product. Whether they do so or not will depend, in large part, on how well-off they feel. This, in turn, will make the profits of high growth firms much more dependent on how well the economy is doing, thus increasing their betas.

Other things remaining equal, an increase in financial leverage will increase the beta of the equity in a firm. Intuitively, we would expect that the fixed interest payments on debt result in high net income in good times and low or negative net income in bad times.
Higher leverage increases the variance in net income and makes equity investment in the firm riskier. If all the firm's risk is borne by the stockholders (i.e., the beta of debt is zero) and debt has a tax benefit to the firm, then,

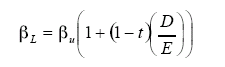
where

ß_L = Levered Beta for equity in the firm
ß_u = Unlevered beta of the firm (i.e., the beta of the firm without any debt)
t = Corporate tax rate
D/E = Debt/Equity Ratio

The unlevered beta of a firm is determined by the types of the businesses in which it operates and its operating leverage. It is often also referred to as the asset beta since it is determined by the assets owned by the firm. Thus, the levered beta, which is also the beta for an equity investment in a firm or the equity beta, is determined both by the riskiness of the business it operates in and by the amount of financial leverage risk it has taken on.

Since financial leverage multiplies the underlying business risk, it stands to reason that firms that have high business risk should be reluctant to take on financial leverage. It also stands to reason that firms that operate in stable businesses should be much more willing to take on financial leverage. Utilities, for instance, have historically had high debt ratios but have not had high betas, mostly because their underlying businesses have been stable and fairly predictable.

The spreadsheet levbeta.xls can be used to calculate the levered and unlevered beta. Current beta in the calculation is the historic beta value. The betas for UK companies can be found on the Monyeam website in the Company Zone area by entering the EPIC code of the desired company.

Breaking down betas into their business risk and financial leverage components provides us with an alternative way of estimating betas in which we do not need past prices on an individual firm or asset.
To develop this alternative approach, we need to introduce an additional property of betas that proves invaluable. The beta of two assets put together is a weighted average of the individual asset betas, with the weights based upon market value. Consequently, the beta for a firm is a weighted average of the betas of all the different businesses it is in.

We can estimate the beta for a firm in five steps.

1. We identify the business or businesses the firm operates in.
2. We find other publicly traded firms in these businesses and obtain their regression betas, which we use to compute an average beta for the firms, and their financial leverage.
3. We estimate the average unlevered beta for the business, by unlevering the average beta for the firm by their average debt to equity ratio. Alternatively, we could estimate the unlevered beta for each firm and then compute the average of the unlevered betas. The first approach is preferable because unlevering an erroneous regression beta is likely to compound the error.
   (
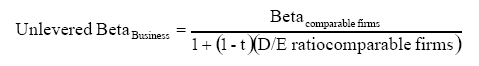
4. To estimate an unlevered beta for the firm that we are analyzing, we take a weighted average of the unlevered betas for the businesses it operates in, using the proportion of firm value derived from each business as the weights. If values are not available, we use operating income or revenues as weights. This weighted average is called the bottom-up unlevered beta.
   
   where the firm is assumed to operating in k different businesses.
5. Finally, we estimate the current market values of debt and equity of the firm and use this debt to equity ratio to estimate a levered beta. The betas estimated using this process are called bottom-up betas.

At first sight, the use of bottom up betas may seem to leave us exposed to all of the problems we noted with regression betas. After all, the betas for other publicly traded firms in the business are obtained from regressions. Notwithstanding these bottom up betas represent a significant improvement on regression betas for the following reasons.
While each regression beta is estimated with standard error, the average across a number of regression betas will have much lower standard error. The intuition is simple. A high standard error on a beta estimate indicates that it can be significantly higher or lower than the true beta. Averaging across these errors results in an average beta that is far more precise than the individual betas that went into it. In fact, if the estimation errors on individual firm betas are uncorrelated across firms, the savings in standard error can be stated as a function of the average standard error and the number of firms in the sample.

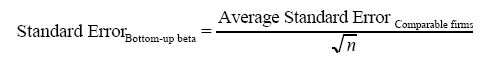
where n is the number of firms in the sample. Thus, if the average standard error in beta estimates for software firms is 0.50 and the number of software firms is 100, the standard error of the average beta is only 0.05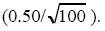

A bottom-up beta can be adapted to reflect actual changes in a firm's business mix and expected changes in the future. Thus, if a firm divested a major portion of its operations last week, the weights on the businesses can be modified to reflect the divestiture. The same can be done with acquisitions.
In fact, a firm's strategic plans to enter new businesses in the future can be brought into the beta estimates for future periods.

Firms do change their debt ratios over time. While regression betas reflect the average debt to equity ratio maintained by the firm during the regression period, bottom-up betas use the current debt to equity ratio. If a firm plans to change its debt to equity ratio in the future, the beta can be adjusted to show these changes. Finally, bottom-up betas wean us from our dependence on historical stock prices. While we do need these prices to get betas for comparable firms, all we need for the firm being analysed is a breakdown of the businesses it is in. Thus, bottom-up betas can be estimated for private firms, divisions of business and stocks that have just started trading in financial markets.

While the idea behind bottom-up betas is fairly simple, there are several computational details that are deserving of attention.

1. Defining Comparable firms: First, we have to decide how narrowly we want to define a business.
   Consider, for instance, a firm that manufactures entertainment software. We could define the business as entertainment software and consider only companies that primarily manufacture entertainment software to be comparable firms. We could go even further and define comparable firms as firms manufacturing entertainment software with revenues similar to that of the company being analysed.
   While there are benefits to narrowing the comparable firm definition, there is a large cost. Each additional criterion added on to the definition of comparable will mean that fewer firms make the list and the savings in standard error that comprise the biggest benefit to bottom-up betas become smaller.
   A common sense principle should therefore come into play. If there are hundreds of firms in a business, as there are in the software business, you can afford to be more selective. If there are relatively few firms, not only do you have to become less selective, you might have to broaden the definition of comparable to bring in other firms into the mix.
2. Estimating Betas: Once the comparable firms in a business have been defined, you have to estimate the betas for these firms. While it would be best to estimate the regressions for all of these firms against a common and well diversified equity index, it is usually easier to use service betas that are available for each of these firms. These service betas may be estimated against different indices. For instance, if you define your business to be global telecommunications and obtain betas for global telecomm firms from Bloomberg, these betas will be estimated against theirlocal indices. This is usually not a fatal problem, especially with large samples, since errors in the estimates tend to average out.
3. . Averaging Method: The average beta for the firms in the sector can be computed in one of two ways. We could use market-weighted averages, but the savings in standard error that we touted in the earlier section will be muted, especially if there are one or two very large firms in the sample. We could estimate the simple average of the betas of the companies, thus weighting all betas equally. The process weighs in the smallest firms in the sample disproportionately but the savings in standard error are likely to be maximised. There is also the issue of whether the firm being analysed should be excluded from the group when computing the average. While the answer is yes, there will make little or no difference in the final estimate if there are more than 15 or 20 comparable firms.
4. Controlling for differences: In essence, when we use betas from comparable firms, we are assuming that all firms in the business are equally exposed to business risk and have similar operating leverage. Note that the process of levering and unlevering of betas allows us to control for differences in financial leverage. If there are significant differences in operating leverage û cost structure û across companies, the differences in operating leverage can be controlled for as well. This would require that we estimate a business beta, where we take out the effects of operating leverage from the unlevered beta.
   

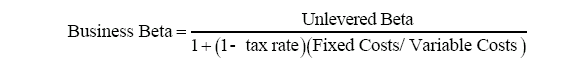
   Note the similarity to the adjustment for financial leverage; the only difference is that both fixed and variable costs are eligible for the tax deduction and the tax rate is therefore no longer a factor. The business beta can then be relevered to reflect the differences in operating leverage across firms. betas.xls: